Mastering The FOIL Method With Three Terms: A Comprehensive Guide
Have you ever wondered how to multiply binomials with three terms effectively? The FOIL method with three terms is a powerful tool in algebra that simplifies complex equations and helps you solve problems faster. Whether you're a student struggling with algebra or a math enthusiast looking to refine your skills, understanding this method can make all the difference. FOIL stands for First, Outer, Inner, Last, and it’s traditionally used for multiplying two binomials. However, when extended to three terms, it opens up a whole new world of possibilities.
At its core, the FOIL method with three terms is about breaking down equations into manageable parts. By focusing on each pair of terms systematically, you can tackle even the most challenging problems with ease. This method is widely used in algebra, calculus, and other advanced mathematics, making it an essential skill for anyone pursuing a STEM-related field. The process not only simplifies calculations but also enhances your logical thinking and problem-solving abilities.
So, why is mastering the FOIL method with three terms so important? Beyond its practical applications, it builds a strong foundation for understanding more complex mathematical concepts. From expanding polynomials to simplifying expressions, this technique is a cornerstone of algebraic success. In this article, we’ll explore the intricacies of the FOIL method with three terms, provide step-by-step guidance, and answer common questions to help you become a math wizard. Let’s dive in!
Read also:Zodiac Sign For January 25 Everything You Need To Know
Table of Contents
- What is the FOIL Method with Three Terms?
- How Does the FOIL Method Work?
- Can the FOIL Method Be Applied to Three Terms?
- Step-by-Step Guide to Using the FOIL Method
- Common Mistakes to Avoid When Using the FOIL Method
- Why is the FOIL Method Important in Algebra?
- How Can You Practice the FOIL Method Effectively?
- Frequently Asked Questions About the FOIL Method
What is the FOIL Method with Three Terms?
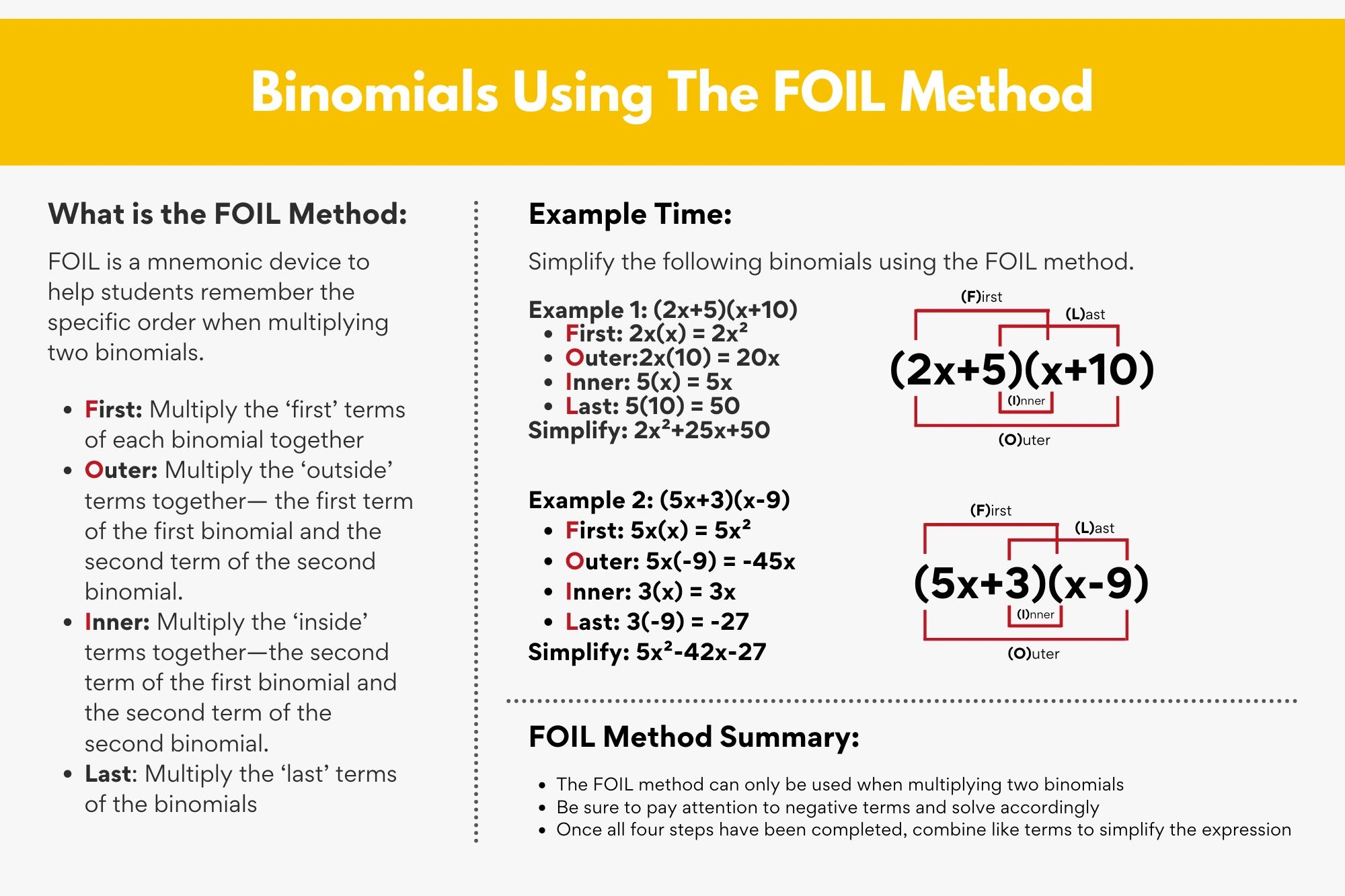
The FOIL method is a mnemonic device used to multiply two binomials. It stands for First, Outer, Inner, Last, referring to the order in which terms are multiplied. While the traditional FOIL method applies to two binomials, extending it to three terms involves a similar systematic approach. This adaptation is particularly useful when dealing with trinomials or more complex algebraic expressions.
When applying the FOIL method with three terms, you essentially break down the multiplication process into smaller, more manageable steps. For instance, if you have three binomials like (a + b)(c + d)(e + f), you would first multiply the first two binomials using the FOIL method and then multiply the resulting polynomial by the third binomial. This ensures that every term is accounted for and no part of the equation is overlooked.
The beauty of the FOIL method with three terms lies in its simplicity and versatility. It can be applied to a wide range of problems, from basic algebra to more advanced mathematical concepts. By mastering this technique, you’ll be better equipped to handle equations that might otherwise seem overwhelming. Let’s explore how this method works in greater detail.
How Does the FOIL Method Work?
To understand how the FOIL method works, let’s break it down step by step. The first step involves multiplying the "First" terms of the binomials. For example, in the equation (a + b)(c + d), the "First" terms are a and c. Multiply these together to get ac. Next, multiply the "Outer" terms, which are a and d, resulting in ad. Then, multiply the "Inner" terms, b and c, to get bc. Finally, multiply the "Last" terms, b and d, resulting in bd.
When extending this method to three terms, the process becomes slightly more intricate. For instance, consider the equation (a + b)(c + d)(e + f). First, use the FOIL method to multiply the first two binomials: (a + b)(c + d). This gives you ac + ad + bc + bd. Next, multiply this resulting polynomial by the third binomial (e + f). Distribute each term in the polynomial to each term in the third binomial to get the final expanded form.
Breaking Down the Steps
- First Terms: Multiply the first terms of each binomial.
- Outer Terms: Multiply the outer terms of the binomials.
- Inner Terms: Multiply the inner terms of the binomials.
- Last Terms: Multiply the last terms of each binomial.
Why is This Method Effective?
The FOIL method is effective because it ensures that every term in the binomials is multiplied by every other term. This systematic approach minimizes errors and makes it easier to track each step of the process. By following the FOIL sequence, you can simplify even the most complex equations with confidence.
Read also:Discover The Largest Domestic Cat Breeds A Comprehensive Guide
Can the FOIL Method Be Applied to Three Terms?
Yes, the FOIL method can indeed be applied to three terms, though it requires a slightly modified approach. When working with three binomials, the key is to use the FOIL method iteratively. Start by multiplying the first two binomials using the traditional FOIL method. Once you have the resulting polynomial, multiply it by the third binomial using the distributive property.
For example, consider the equation (a + b)(c + d)(e + f). First, apply the FOIL method to (a + b)(c + d) to get ac + ad + bc + bd. Then, multiply this polynomial by (e + f) to get the final expanded form. This process ensures that every term is accounted for and no part of the equation is overlooked.
What Are the Benefits of Using the FOIL Method with Three Terms?
- Simplifies Complex Equations: The FOIL method breaks down complicated problems into manageable steps.
- Reduces Errors: By following a systematic approach, you minimize the risk of missing terms or making calculation mistakes.
- Enhances Understanding: This method helps you visualize how terms interact, deepening your comprehension of algebraic principles.
Are There Any Limitations to This Method?
While the FOIL method with three terms is highly effective, it’s important to note that it’s not always the fastest approach for larger equations. In such cases, alternative methods like the distributive property or grouping may be more efficient. However, for smaller equations, the FOIL method remains a reliable and straightforward tool.
Step-by-Step Guide to Using the FOIL Method
Now that we’ve explored the theory behind the FOIL method with three terms, let’s dive into a practical step-by-step guide. This section will walk you through the process of multiplying three binomials using the FOIL method, ensuring you understand each stage of the calculation.
Step 1: Multiply the First Two Binomials
Begin by applying the traditional FOIL method to the first two binomials. For example, in the equation (a + b)(c + d)(e + f), start with (a + b)(c + d). Multiply the "First" terms (a and c) to get ac. Then, multiply the "Outer" terms (a and d) to get ad. Next, multiply the "Inner" terms (b and c) to get bc. Finally, multiply the "Last" terms (b and d) to get bd. Combine these results to form the polynomial ac + ad + bc + bd.
Step 2: Multiply the Resulting Polynomial by the Third Binomial
Once you have the polynomial from Step 1, multiply it by the third binomial (e + f). Distribute each term in the polynomial to each term in the third binomial. For instance, multiply ac by e to get ace, then multiply ac by f to get acf. Repeat this process for each term in the polynomial to get the final expanded form.
Step 3: Combine Like Terms
After completing the multiplication, combine any like terms to simplify the equation further. This step ensures that your final answer is as concise as possible. For example, if you have terms like ace and acf, check if they can be combined or simplified further.
Common Mistakes to Avoid When Using the FOIL Method
While the FOIL method with three terms is a powerful tool, it’s easy to make mistakes if you’re not careful. Here are some common pitfalls to watch out for and how to avoid them:
Mistake 1: Missing Terms During Multiplication
One of the most frequent errors is forgetting to multiply every term in the binomials. To avoid this, double-check that you’ve accounted for all combinations of terms. A helpful tip is to write out each step explicitly and verify your work as you go.
Mistake 2: Failing to Combine Like Terms
After expanding the equation, it’s crucial to combine like terms to simplify the expression. Neglecting this step can lead to unnecessarily complex answers. Always review your final result to ensure it’s as concise as possible.
Mistake 3: Misapplying the Distributive Property
When multiplying the resulting polynomial by the third binomial, ensure that you apply the distributive property correctly. Each term in the polynomial must be multiplied by each term in the third binomial. Skipping this step will result in an incomplete solution.
Why is the FOIL Method Important in Algebra?
The FOIL method is a cornerstone of algebra, providing a systematic way to multiply binomials and polynomials. Its importance lies in its ability to simplify complex equations and make them more approachable. By breaking down problems into smaller, manageable steps, the FOIL method helps students and professionals alike tackle even the most challenging mathematical problems with confidence.
Applications of the FOIL Method
- Expanding Polynomials: The FOIL method is essential for expanding polynomials, a common task in algebra and calculus.
- Solving Equations: It’s often used to solve quadratic equations and other algebraic expressions.
- Real-World Problems: The method has practical applications in fields like engineering, physics, and economics.
How Does It Build Mathematical Skills?
Beyond its practical applications, the FOIL method with three terms enhances logical thinking and problem-solving skills. By encouraging a step-by-step approach, it fosters precision and attention to detail, qualities that are invaluable in mathematics and beyond.
How Can You Practice the FOIL Method Effectively?
Like any skill, mastering the FOIL method with three terms requires practice. Here are some tips to help you hone your abilities and become proficient in this technique:
Tip 1: Start with Simple Problems
Begin by practicing with basic equations involving two binomials. Once you’re comfortable with the traditional FOIL method, move on to more complex problems with three terms. This gradual progression will build your confidence and understanding.
Tip 2: Use Online Resources
There are numerous online tools and tutorials available to help you practice the FOIL method. Websites like Khan Academy offer free lessons and exercises to reinforce your learning.
Tip 3: Solve Real-World Problems
Apply the FOIL method to real-world scenarios to see its practical applications. For example, use it to calculate areas, volumes, or other measurements that involve polynomials. This approach not only reinforces your skills but also highlights the method’s relevance.